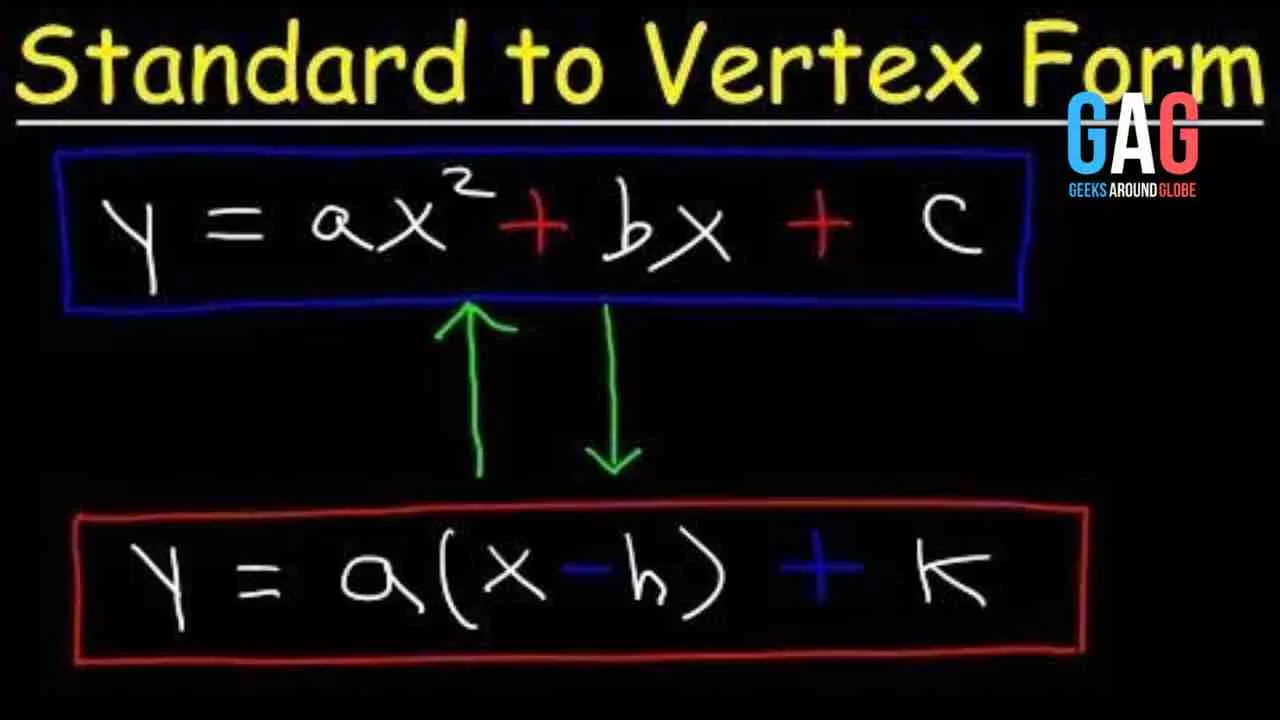
In the field of calculus and analytical geometry, the term vertex corresponds to the coordinates of the parabola that represents the centre value of the quadratic equations. Basically, the coordinates of the vertex are those values that satisfy the vertex form of the quadratic equation. As you are better aware that the standard form is given as:
y=ax2+bx+c
Now the nature of the coefficient of x2 is the factor that decides the shape of the parabola having a vertex over it somewhere. For instance, the free online vertex form calculator by calculator-online.net is the best tool that is intuitively designed to calculate the vertex coordinates.
Vertex of a Parabola:
To better understand the concept of parabola vertex, first you need to get a firm grip over different shapes of parabola.
“A particular ⋃ shaped curly figure that can be used to represent the behaviour of quadratic vertex equation is called parabola”
Cases:
Below we have given various shapes of the parabola and their respective meanings:
- U = It represents the parabola that is opened from the top
- ∩ = it shows the parabola of the equation that is open downwards
- ⊃ = it represents the parabola that is opened towards left
- ⊂ = It shows the parabola that may be opened towards right
The online vertex form calculator will take instants to determine the coordinates of vertex lying on any one of the parabola types aforementioned.
Characteristics of Vertex Form:
Certain properties help us define and understand the behaviour of the vertex of a parabola:
- The vertex represents a curve. It means that where the vertex lies on parabola, the curve actually starts right from there
- Since the vertex shows a sharp bend on the parabola, it derivative will be zero
- The vertex is either relative maxima or minima if the parabola is either opened upward or downward
- The vertex for a right or left opened parabola is neither minima or maxima
- Aa parabola will always intersect its symmetrical axis at the point of bending, that is vertex
Standard Form To Vertex Form Conversion:

Now we will convert to vertex form manually. As you may know that an equation can be represented in the following standard forms:
- Intercept form
- Vertex form
- Standard form
The online vertex form calculator can also perform respective conversions among these kinds of equations but it mostly focuses on vertex equations. So if you are indulged with any difficulty regarding determining the coordinates of the vertex, you should give it a try.
In the vertex form equation of the standard equation which is y=a(x-h)2+k, a complete square is there if you see. Now what you are in need to do is convert this incomplete square to its complete form. There exists a couple of methods to do this, and we will throw a light on both of these!
Method of Completing Square:
For the sake of your ease in understanding the concept, we will resolve an example here that you can also verify by using the best vertex form calculator by calculator online site.
Just make a supposition that we are having the following equation:
y=-3×2-6x-9
The very first step is to make the coefficient of x2 as 1… and in case it is not, we will try to make it as it is! Let us see how!
y=-3×2-6x-9
y=-3(x2+2x+3)
This arrangement is automatically done and in seconds if you input the standard form equation in to the free vertex calculator.
Now the conversion of this equation to the respective vertex form gets started!
- First of all, recognise the coefficient of x which is 2.
Coefficient of x=2
- The next step is to make it half and find the square, such that:
(2/2)2=(1)2=1
- After doing so, go by adding and subtracting the number in the parent equation just after the x term ends:
y=-3(x2+2x+1-1+3)
- Now as you know the formula:
(x+1)2=x2+2x+1
- Following this formula, we will see how the first 3 terms can be joined the same way:
y=-3((x+1)2-1+3)
y=-3((x+1)2+2)
y=-3(x+1)2+6
The above expression is just as same as a vertex equation. So this is the final result. For the sake of your satisfaction, you may yourself utilise the vertex form calculator to verify the results.
Formula Method:
The coordinates of vertex that are h and k respectively, are very helpful in determining the vertex form of the equation. And if you scroll a little up and see the completing square method, you will notice that these parameters are not calculated in that method. So the formula method will let you determine these values as well. And for maintaining the accuracy, the vertex form calculator also considers this formula to make calculations.
h=-b/2a
Once you calculate its value, put it in the equation below to determine the k value as well:
k=ah2+bh+c
Example:
Determine the vertex form equation of the following standard equation given:
y=9×2+6x-7
Solution:
Here we have:
h=-b/2a
h=-6/2*9
h=-6/18
h=-1/3
Now we have:
k=ah2+bh+c
k=9(-1/3)2+6(-1/3)+(-7)
k=9(1/9)-6/3-7
k=1-2-7
k=1-2-7
k=-10
For instant verification of results, you can put in the coefficients in the vertex form calculator and get immediate results.
Faq:
How To Find The Focus of Parabola Using Its Vertex?
Suppose we have a point as follows:
Vertex=(h, k)
Its focus can be determined as:
Parabola Focus=>y=a(x-h)2+k=>(h, k+(1/4a))
Parabola Focus=>x=a(y-h)2+k=>(h+(1/4a), k)